I can see some chat on internet about the operational amplifier gain bandwidth product. People are interested in having a better understanding of this parameter, as it appears in any op amp datasheet and it is used in many articles and books. In this article I will describe this parameter and show you an example with Analog Devices’ ADA4004, which is a precision amplifier.
The Gain Bandwidth Product describes the op amp gain behavior with frequency. Manufacturers insert a dominant pole in the op amp frequency response, so that the output voltage versus frequency is predictable. Why do they do that? Because the operational amplifier, which is grown on a silicon die, has many active components, each one with its own cutoff frequency and frequency response. Because of that, the operational amplifier frequency response would be random, with poles and zeros which would differ from op amp to op amp even in the same family. As a consequence, manufacturers thought of introducing a dominant pole in the schematic, so that the op amp response becomes more predictable. It is a way of “standardizing” the op amp frequency response. At the same time, it makes the op amp more user friendly, because its stability in a schematic becomes more predictable.
The dominant pole will make the op amp behave like a single-pole system, which has a drop of 20 dB for every decade of frequency, starting with the cutoff frequency. Such a pole is made with a reactive element, usually a capacitor. The choice of a capacitor on the op amp die is because of the manufacturing process. A capacitor is easier to be grown on silicon, as opposed to an inductor. However, the capacitor value depends on its area, and since the die area is at a premium, capacitors can only be very small, in the picofarads range. Since the pole is made with an RC time constant, we need a large resistor to bring the cutoff frequency at low values, hertz, or tens of hertz. Without going into details, op amp manufacturers achieve these high resistors with active components, like the input resistance seen in a transistor base.
Having said that, the gain bandwidth product shows that the product between the op amp gain and frequency, in any point of the frequency response, is a constant. We can always calculate the bandwidth with the following formula.
| (1) |
In the case of ADA4004, the gain bandwidth product is 12 MHz. This means that, at a gain of one, the bandwidth is 12 MHz, and at the maximum open-loop gain of 500000, the bandwidth is 12 MHz divided by 500000, which is 24 Hz. This is the op amp open-loop cutoff frequency.
The mathematical model of the gain bandwidth product is described by the following single-pole function:
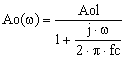 |
(2) |
where ω is the variable, or the function argument, which is 2π times frequency, fc is the op amp cutoff frequency, Aol is the open-loop gain at DC, and j is the imaginary unit.
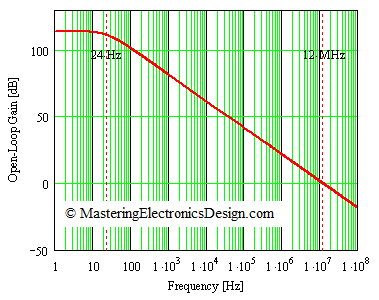
Figure 1 shows a Mathcad plot of this function. The gain starts at low frequencies at 20 log(500000) = 114 dB and then rolls off down to 0 dB. The markers show the cutoff frequency at 24 Hz and 111 dB gain, and the unity-gain frequency at 12 MHz and 0 dB gain. Why is the gain 111 dB at 24 Hz? Because the cutoff frequency is at -3 dB, which means that the corresponding gain is 114 dB – 3dB = 111 dB.
Figure 1
For simplicity and clarity, the gain is always shown in dB. One can see that, at low frequencies, the gain is 20 log(500000) = 114 dB. Starting with the cutoff frequency of 24 Hz, the gain rolls off at a rate of 20dB/decade until 12 MHz, where the gain is 1, or 0 dB. This theoretical graph is almost identical with the ADA4004 open-loop gain characteristic as shown in its datasheet (Rev. F) Figure 14.
The advantage of using the gain bandwidth product parameter lays in the fact that we can always predict the op amp bandwidth for a certain gain.
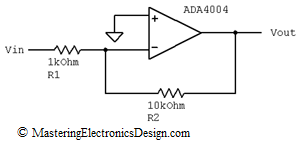
As an example, Figure 2 shows an inverting amplifier with ADA4004.
Figure 2
The gain is set by the ratio between R2 and R1 (go to this article How to Derive the Inverting Amplifier Transfer Function to see why). The resistor ratio is 10, so the bandwidth is 12 MHz divided by 10 which is 1.2 MHz. This result matches the ADA4004 closed-loop gain characteristic, which is shown in the same datasheet, at Figure 19.





Hi Adrian,
your series about opamp applications is appreciated, however, I have some comments to your gain-bandwidth justification:
Quote:
“At the same time, it makes the op amp more user friendly, because its stability in a schematic becomes more predictable.”
More predictable?
I think, the only reason for a single pole roll-off is to ENSURE stability for all feedback factors. I doubt if “standardizing” of the opamps behaviour is the main goal. That`s really not the point!
I hope that you are going to write something about stability issues in the future – in particular, which options exist to use opamps that are NOT universal-compensated even in unity gain applications.
Regards
Lutz
Hi Lutz, thank you for your visit and for taking your time to comment. We are in agreement here. When I wrote “more predictable” I was thinking at op amp stability and bandwidth expectancy for gains down to unity. I appreciate you making a point for clarity.
Yes, I plan to write more about stability, and about op amps that do not have this compensation.
Hi Adrian, in the above example the maximum gain is 114dB and at cutoff frequency it becomes 111dB. The gainbandwidth product is given to be 12Mhz because at 0dB frequency is 12Mhz. Then shouldnt the cutoff frequency be 33.82Hz ? Because 111dB=354813.38(v/v) and 354813.38*33.82= approx12Mhz
No, it is not 33.82 Hz, and here is why:
Take a look at the following image, where I zoomed into the cutoff frequency.
If you use the gain-bandwidth product to calculate the cutoff frequency, it will give you the frequency at the intersection of the two asymptotes: the plateau and the roll off. That is why the cutoff frequency is also called the corner frequency. If you use the gain bandwidth product to find the cutoff frequency, you need to use the gain at DC, in this case 114 dB. It is an approximation. In reality, there are no corners in Nature, since our world is analog. Instead of a corner, there will always be a roll off. So, you calculate the cutoff frequency with the DC gain, 114 dB, and if you want to know the actual gain at the frequency you just calculated, 24 Hz, subtract 3 dB from the DC gain, in this case 111 dB.
Another way to calculate the actual gain at 24Hz is to use the exact mathematical relation of the gain, given in the article, equation (2). If you calculate the absolute value of the gain at 24 Hz, which is |Ao(2*pi*24Hz)|, you will find a gain of 353553.39. In dB that is 110.969 dB.
Shouldn’t the gain from the example be 11 instead of 10 (because it’s a non-inverting amplifier)?
Nice article, very informative 🙂
Correct, the link points to the wrong figure. It was supposed to be an inverting amplifier. I corrected that. Thank you for discovering that error.
what is the gain bandwidth product of an op-amp, when a negative feedback is used,
remains same or unity?
The Gain Bandwidth Product remains the same. It is an Op Amp parameter, found in its datasheet. What changes is the end circuit bandwidth, which depends on the negative feedback level. The article explains the relationship between bandwidth, negative feedback (or gain) and the gain bandwidth product.
Adrian:
Can you please comment on a test result that I am getting, in light of the gain bandwidth product of an op-amp.
I am using an opamp (LTC6244) with GBW = 35 MHz in a Sallen Key filter application. Input is a square wave of frequency 4MHz, and 80% duty cycle. Measured output is less than the estimated output. At 50% duty cycle, output is the same as what I estimated. It appears that this is due to GBW limitations. Can you please explain the mechanism.
Santhi, Any electronic system has a bandwidth and, because of that, it will always limit the input signal spectrum. You say your LTC6244 has a GBW of 35MHz, but the overall circuit might have a lower bandwidth, depending on the circuit gain. The bandwidth is restricted even more because you designed a filter.
Now let’s look at the input signal. You say it is a square wave with a frequency of 4 MHz and 80% duty cycle. Well, in this case, the signal has a steep rise and fall-time, or sharp edges. To achieve that, it needs many harmonics in its spectrum. Fourier transform shows that, to have that perfect square in a pulse, we need an infinity of superimposed sinewave signals. So, your input signal has a large spectrum, which is cut by your limited bandwidth.
A rule of thumb is to have at least 10 unattenuated harmonics passing through your circuit to have a good approximation of the input signal. Since you designed a filter, you obviously want to modify the shape of the signal. At 4 MHz, the signal has the following harmonics: 4, 8, 12, 16, 20, 24, 28, 32, 36, … MHz. The Op Amp by itself, with a gain of one, will pass through just 8 unattenuated harmonics. If the Op Amp has a gain of 2 as an example, the bandwidth will be 35/2 = 17.5 MHz, so there will be just 4 unattenuated harmonics passing through your circuit. To verify your results, you really need to calculate the Fourier transform components and then add them with their corresponding phases.
Quality maintained site ! Great and Thank all the team
Thank you for your comment. It is not a team. It is just me.
plz Adrian S. Nastase can you comment or write any thing about CMOS OP AMP and some parameter for it!!!
CMOS Op Amps improved over time and, today, they have better specs than they used to. The main advantage of CMOS Op Amps is having low input bias currents, down to picoamps level. They also may have low power consumption, provided that the OP Amp is not designed for high frequency operation. Still, bipolar Op Amps are better in handling high frequencies, although modern CMOS Op Amps started to have good gain-bandwidth product.
When designing with Op Amps, I usually look at specifications, and make my choice not based on technology but on my needs for the design at hand. With CMOS one can expect higher low frequency noise (the so called 1/f noise). Voltage offset used to not be stellar, but in the recent years manufacturers used trimming techniques at the wafer level to compensate for offset. There is also the BiCMOS technology which was designed to combine the advantages of both bipolar and CMOS processes.
So, which Op Amp to use? Choose a few that you feel are close to what you need for your design and then narrow down your search by comparing their specs. That’s what engineers usually do, a balancing act between price, performance and settling on what’s important and what not when choosing an electronic component.
For more information on this subject I suggest you read Analysis and Design of Analog Integrated Circuits by Paul R. Gray and others. This book has excellent info regarding CMOS Op Amps. Another good book is Introduction to CMOS OP-AMPs and Comparators by Roubik Gregorian.
Hi, I have an op amp with the specs DC Voltage Gain 10000 (RL=500 ohm),
Output + , – 10V @ 20mA, Frequency for full output 100 kHz (min) , Slew rate 6V/microsec (min) What wold be the feedback or gain and max input V for this op amp
thanks
To answer your question I also need to know the gain-bandwidth product. Bandwidth and slew rate are different. An Op Amp can have great bandwidth but poor slew rate.
hi,
You have a very useful page here. Just wanted to ask a simple question… How does the GBW and slew rate limit the operation of the amplifier in practice? :s Im a bit confused.
thank you.
GBW limits the bandwidth of the amplifier. In closed loop, as the amplifier gain increases, it’s bandwidth decreases. As such, GBW may limit the signal bandwidth if we try to amplify a signal with frequency components outside the amplifier bandwidth.
Slew-rate may distort the signal. At higher amplitudes, the signal behavior in time might be linearized by slew-rate. you can see this if the signal has sudden changes in amplitude. A slew-rate limiting amplifier will simply output a ramp instead of the sudden change, distorting the input signal.
Thanks for this resource, I want to select the components to make my own HV amplifier for a function generator, and the series on op-amps has been useful in gaining some understanding. Given that I don’t have a background on electronics, it has proven a bit difficult. I have been looking at AD’s chips that have test boards made for them, as this might make the process a bit more simple.
You’re welcome JR. Let me know how it goes.
hi
why we connect capacitor between op amp terminals?
You need to tell me more to give you an answer. What capacitor are you referring to? Which terminals?
Hi Adrian,
I am doing a work on fully differential Negative feedback op-amp with capacitive divider configuration.I have some questions and confusions, can u plz clarify?
What is the difference between Closed loop gain and open loop gain, and are they dependent to each other?
How can we calculate the unity gain frequency if I have a 3-dB frequency of 100Hz and closed loop gain of 40dB?
Does the feedback factor (BETA) has importance with respect to any other parameters?
How does it will help in finding the closed transfer function of the system assuming the op-amp as a single pole system?
I will be obliged for your help.
The answers cannot fit in this comment space, so I will post an article. Come back soon or subscribe.
Update: I posted the answers here: Open-loop, Closed-loop and Feedback Questions and Answers.
high am designing a non-inverting op-amp with a gain of 30dB and a Lower cut-off frequency=22.38721Hz
Upper cut-off frequency=5011.872Hz
i have an input high-pass filter with R=22Kohms, C=2.2uF and an output low-pass with R=2.2ohms, C=0.47uF
are you able to explain to me how this achieves the human hearing range of 20Hz – 20kHz. thanks
Richard, your components do not match the filter values you described, so I will ignore the resistors and capacitors for now. An amplifier with a high pass filter of 22 Hz will pass all the frequencies above the 22 Hz, so it can be considered a human hearing amplifier at low frequencies.
At high frequencies, since it has a low pass filter of 5 kHz, it will will start attenuating frequencies above 5 kHz at a rate of 20 dB per decade. As such, at 20 kHz the amplifier gain will be about 7. Most likely this amplifier is designed to achieve a human hearing compensation above 5 kHz. If you look at ear sensitivity graphs you can find on internet, the ear has a higher sensitivity above 5 kHz. As an example, look at graph 1, in this article, http://www.audioholics.com/education/acoustics-principles/human-hearing-lamplitude-sensitivity-part-1. The human ear sensitivity increases above 5 kHz, so having an amplifier with the gain that decreases above 5 kHz will create an overall gain plateau between 5 and 20 kHz. So, yes, this amplifier can be a human hearing amplifier based on the data you gave me.
is it possible to achieve high gain bandwidth product using unipolar supply cmos opamp?
CMOS op amps came a long way and now they have good bandwidth, fast slew rate, low cost, and unipolar power supply. Take a look at Analog Devices’s ADA4891 family. It has 220MHz GBW product, 170 V/us slew-rate, it is rail-to-rail, and can be powered from a single 5V supply.
Hi Adrian,
I’m looking forward to amplifiy a signal with a frequency of 125 KHz. The gain i need has to be at least 40 db. As i have understood, I might need an op Amp with GBP=125 KHz*100=12.5 MHz at least. Correct me if i’m wrong. Also, There are some nanowatt op amp out there. I’m designing an ultra-low power circuit and I’m intending to use them. However, i believe the input signal frequency has to around few Hz in order to achieve the gain I mentioned since these op amp GBP is around 10 KHz.
Correct. You need an amplifier with GBP >= 12.5 MHz. I doubt you will find a nanowatt op amp with this bandwidth. You can find ultra low power op amps, with the quiescent current of 250 uA and +/- 1.25 V supplies, like the Texas Instruments’ OPA835. The higher the bandwidth, the higher the power consumption. That’s because the op amp internal components, like resistors, have to have low value, to push the amplifier poles to higher frequencies.
what is the significane of band gain width product?
If your question is about the gain bandwidth product, the second paragraph of this article https://masteringelectronicsdesign.com/an-op-amp-gain-bandwidth-product/ gives you the answer.
Nice explaination, but why gain bandwidth product remains constant.??
It is the normal behavior of a single pole amplifier. After the cutoff frequency the gain drops at 20 dB/decade, so the product between the gain and bandwidth in each point of the curve is constant. I will post a small article showing how this can be proven mathematically.
=====================================
Update: You can find this article here: Why is the Op Amp Gain-Bandwidth Product Constant?
why gain b/w product remains constant with the introduction of negative feedback?
Dear Adrian,
could you please explain how to calculate the bandwidth of an op amp with two or more break frequencies from a given dB/frequency log graph.thanks
Eric, even if the amplifier has more break frequencies, the bandwidth is determined the same as in this article. In open loop, the bandwidth is the frequency point where the gain becomes 0.707 of the DC gain. If you close the loop, you know your gain. Mark the gain on the y axis, draw a horizontal line through that point, and the intersection with the op amp graph shows the closed loop bandwidth.
the amplifier gain is 82.3 dB. the unit GainBandWidth is 102 MHz then what is the Bandwidth..??
if bandwidth is 20000Hz then relation among all is true…???
I don’t understand this. Please reformulate your question.
This sounds like homework, so I will not solve it for you. I can tell you how to solve it yourself. Transform the gain from dB to gain ratio, to find the closed-loop gain. Then use equation (1) in this article to find the bandwidth.
An operational amplifier has a norminal gain of 100 dB. The gain is constant 1-10 Hz and reduces by 20 dB per decade above 10 hz
(i) Calculate the gain/bandwith product
(ii)Determine the bandwidth of the amplifier if the closed loop voltage gain is 40 dB?
This sounds like homework and I will not do it for you. All I can say is that this article helps you to calculate both answers. The problem gives you the corner frequency and the nominal gain, as well as the drop of 20 dB/decade. Read the article and apply the equations.
i did an experiment to measure the bandwidth and the slew rate of an op amp but ineed an conclusion
Is there a question? Tell me more.
hi Adrian,
Firstly i have very little knowledge of electronics. so sorry for it if anything conflicts or mistakes done by me.
I am trying to generate sine waves with arduino uno connected with R2R DAC . i have generated sine waves upto 111kHZ with 13 samples per wave i.e. sampling rate of around 1.5 MHz.
Now i want to drive some piezoelectric wafers using these sine waves. But problem is that impedance of R2R DAC is very high. So, i came to know about opamps.
Now i am confused that my sampling rate is 1.5 MHz and sine wave freq. is 111kHz. Then what should be the Gain bandwidth of opamp. is it should equal to sampling rate or it should equal to a frequency of sine waves?
can u please help me to understand what is exactly this GB frequency means ?
is it frequency of sine waves or frequency of sampling ?
i am waiting for your reply
Mandar, I just saw your comment. You want to let the 111kHz signal go through and block the sampling frequency, since the low frequency signal is what you need in your application. Usually op amps have high bandwidth. It will be impossible to find one that has a bandwidth between 111kHz and 1.5MHz. So, the practical approach is to get an op amp with a bandwidth that covers your low frequency generated signal and include components to filter the sampling frequency. In other words, you need an op amp with a low pass filter of, say, 120 kHz.
Hi adrian.
I want to build a low-pass filter with a cut-off frequency 150khz using op-amp.
What is the UGB requirement of the op-amp and why?
Please suggest some proven op-amps.
Thanks in advance.
BR,
Nagaraju G
HI adrian
I want to know is gain bandwidth product is defined only for single pole amplifiers?
and also what if amplifier has bandpass magnitude graph?
It is commonly used for one-pole compensated amplifiers.
Regarding the second question, if this is a one-pole bandpass then, yes, it applies.
Please calculate the gain of the op-Amp if no feedback is present
a) as a unit less than ratio
B) as a logarithmic unit
C)at -3dB
This sounds like a homework, so I am not going to do it for you. Hint: The answers are in this article.
When shopping for op amps there’s a wide range of GBWP available. It seems like in general the higher the better. Is there any reason or case to intentionally use a low GBWP?
Thanks for the articles.
In the past, if you needed a precision op amp you would have to give up in bandwidth. Nowadays, precision op amps are faster and faster. So, if GBWP is not a concern for your application, then look at the other specs. Choose an op amp that is suitable for your task at hand.
Hi,
Question regarding datasheet specs for GBP.
Usually, i observed that op amp datasheets have gain Bandwidth products with test conditions of Av and RL. I believe this means that the product was characterized on these constant gain and RL values which GBP can be calculated by frequency sweep until a -3db is observed on the gain. At this point, the product of the gain and freq is the GBP.
My problem is, i also encountered another datasheet where the test condition is a frequency. So this must also mean that they supply the product with constant freq signal and measure the gain, but how could you apply the -3db requirement to determine that the product is already in roll off if we dont have a constant gain? How do we measure the GBP with constant test frequency?
Please point to the datasheet that has frequency as a parameter for GBP.
For GBP of a OP amp, what is the gain refers to: current gain, voltage gain or power gain? OP amp generally have a high input impedance. So it has a very high current gain. Does this mean it would have a very low band-width?
The op amp by itself is a voltage amplifier with high gain. So, GBP refers to voltage gain.