A recurring conversation I have usually starts with two questions: Why is the op amp gain-bandwidth product constant? And, how can we prove that?
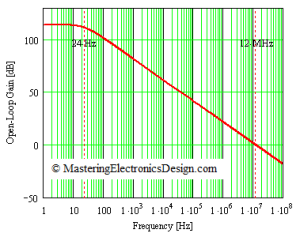
The questions refer to the gain-bandwidth product behavior of an op amp after the cutoff frequency. As I showed in this article, MasteringElectronicsDesign.com: An Op Amp Gain Bandwidth Product, the gain bandwidth product describes the op amp gain dependency on frequency. The open loop graph is shown in Figure 1.
Figure 1
Also, in that article, the mathematical expression of the graph is shown as
| (1) |
where ω is the variable, or the function argument, which is 2π times frequency, ωc is the op amp cutoff frequency, or 2π times fc, Aol is the open-loop gain at DC, and j is the imaginary unit.
This is a one pole system, therefore it drops constantly at 20 dB/decade after the cutoff frequency, as the graph shows. This means that the amplitude of a signal that goes through this op amp decreases 10 times for each frequency decade. The amplitude decreases as the frequency increases showing a constant gain-bandwidth product.
We can prove that the gain bandwidth product is constant by first finding the absolute value of the gain and then multiply it by ω. Equation 1 is a complex number, so we need to apply some complex numbers transformation to find its absolute value. Let’s multiply the function with the conjugate of 1+jω/ωc.
| (2) |
The graph shows the absolute value of Ao(ω). To determine the absolute value, we need to write the square-root of the sum of the real part squared and the imaginary part squared.
| (3) |
As ω increases, the ratio ω/ωc becomes quickly large, so 1 can be neglected. The absolute value of Ao(ω) becomes linear after ω > 3.3 ωc. As such, |Ao(ω)| can be written as
| (4) |
where with Gain(ω) I noted the absolute value of the gain which depends on frequency.
The gain-bandwidth product (GBW) is calculated by multiplying the absolute value of the gain with ω.
| (5) |
and so,
| (6) |
which shows that the gain-bandwidth product is a constant, because it is a product between two constants: the op amp open-loop gain and the corner frequency.



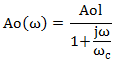
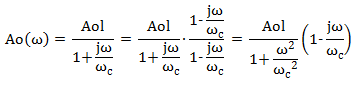



nice blog
Nice proof. Thank you.
Adrian,
Can you explain how you derived equation 1?