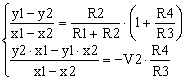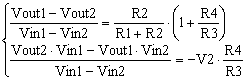The common-mode voltage can bring errors in the differential amplifier applications. What is the common-mode voltage? The common-mode voltage is the voltage level common to both inverting and non-inverting inputs of the differential amplifier. In many applications, the differential amplifier is used to amplify the difference between two voltages, for later processing, or to isolate a signal from common-mode noise, or to amplify a signal that rides on top of some large voltage level. If the common-mode voltage is not rejected, it appears as an error at the amplifier output.
It is customary to consider the common-mode error as being negligible, based on the high Common-Mode Rejection Ratio (CMRR) of the operational amplifiers. This is not always the case. Once the electronics designer connects resistors around this amplifier, in a differential configuration, the common-mode error starts to be significant.
The common-mode voltage Vcm and the differential voltage Vd are shown in the group of equations (1).
| (1) |
Why these expressions? How was Vcm defined like that and why? We will start by looking at the significance of each input voltage in the differential amplifier.
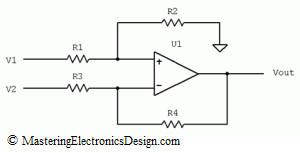
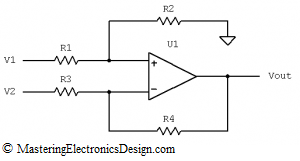
Looking at Figure 1, V1 is the input voltage between R1 and ground, while V2 is the input voltage between R3 and ground.
Figure 1
As we saw in MasteringElectronicsDesign.com: The Differential Amplifier Transfer Function, the signal at the amplifier output is as follows:
| (2) |
If we arrange this equation differently, as in (3),
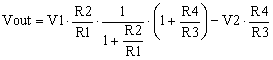 |
(3) |
one can see that, in the unique case in which
| (4) |
the circuit amplifies the difference of the input signals, V1-V2. In other words,
| (5) |
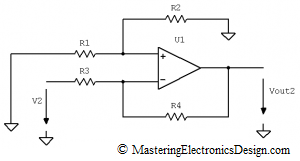
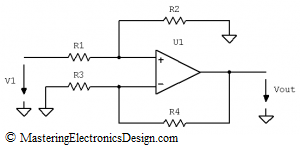
So, which is the common-mode voltage? In order to give you an answer, let’s rearrange the input signals as in Figure 2.
Figure 2
It should be clear now that, when the ratio of the resistor pairs is equal, V2 contribution to the output signal is zero. This can also be seen from equation (2) written differently, as in (6). In equation (6), I grouped the terms so that two main signals are shown: the difference V1-V2 and V2.
 |
(6) |
How did I arrive at this equation? It can be done in two ways: mathematically, using simple algebra methods, or, by using the Superposition Theorem.
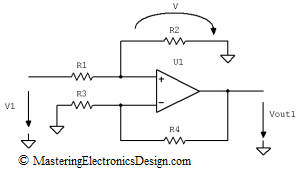
Using the Superposition Theorem is easier, because we can consider that there are two voltage sources in the circuit in Figure 2. One source is V1-V2 and the other one is V2. Based on the Superposition Theorem if we take out one source, V2, and replace it with a wire, we find the first term of equation (6). Indeed, when R3 is connected to ground, the amplifier in Figure 2 becomes a non-inverting amplifier. As I showed in a previous article, MasteringElectronicsDesign.com: The Differential Amplifier Transfer Function, Vout1 is the voltage at the non-inverting input times the gain given by R4 and R3.
| (7) |
With Vout1 I noted the output voltage when V2 is zero.
By rearranging
 we arrive at the first term of equation (6).
we arrive at the first term of equation (6).
The second term of equation (6) is the output voltage when V1-V2 is made zero. In this case the amplifier in Figure 2 is a differential amplifier with the same voltage, V2, at both inputs. Hence, the second term of equation (6).
Equation (6) is important because it shows the common-mode error. Since the circuit amplifies the difference V1-V2, this signal appears as riding on top of V2. Hence, V2 can be seen as a common-mode voltage. If the resistor ratios are rigorously equal, the second term in equation (6) is zero. If they are not, the same term will show up at the amplifier output as an error. This is the common-mode voltage error.
How big is this error and why should the electronics designer be concerned about it?
Let’s consider that the ratio of the resistors is equal, as in equation (4), and that only R2 has a tolerance t which can be positive or negative, but smaller than 20%. In other words:
| (8) |
For resistors, this is a practical assumption. Examples of usual resistor tolerances are 0.1%, 1%, 10%, 20%. In my example R1, R3 and R4 are ideal resistors, with 0 tolerance, while R2 has a tolerance of, say, 10% which I noted with t. This creates a mismatch in the resistor ratios R2/R1 and R4/R3 , so that the common-mode voltage V2 appears at the differential amplifier output, scaled by a factor dependent on the tolerance t. This voltage level is the common-mode error.
To calculate this error, let’s write the common-mode portion of the differential amplifier output by taking into consideration the tolerance t of resistor R2,
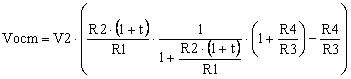 |
(9) |
where with Vocm I noted the common-mode voltage at the differential amplifier output. Since the signal of interest is the difference V1-V2, the common-mode error at the differential amplifier output is Vocm.
After calculations, and using (4), Vocm becomes
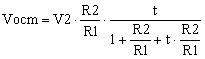 |
(10) |
We can consider that t·R2/R1 is small compared with the ratio R2/R1 which determines the gain of the amplifier. Also, for gains larger than 10, the value of 1 in the denominator can be neglected. Therefore, the common-mode error Vocm is
| (11) |
Equation (10) shows that, if one resistor, R2, has a tolerance other than zero, there is a significant error at the differential amplifier output, which is approximately the common-mode voltage times that tolerance.
As an example, if V2 = 10V, V1 = 10.1V, and
the circuit in Figure 1 amplifies the difference between these two signals, so that the output is 2V.
However, if R2 has a tolerance of +10%, the error at the circuit output is Vocm = 10V·0.1 = 1V. As a result, the differential amplifier output will be the sum of the differential output of 2V and the error of 1V, which makes 3V. The error of 1V is significant.
If R2 has a tolerance of 0.1%, the error is 10mV, which can be considered negligible in some applications. That is why the usual recommendation is to have either highly matched resistors for the differential amplifier, or resistors with 0.1% or even 0.05% tolerance.
The same logic is valid for V1 that can be viewed as the common-mode voltage, while the circuit amplifies the negative difference -(V1-V2). In the next part I will show that the convention for the common-mode voltage is
and also the reason why this is the preferred method.